Perfection?

One of the most famous baseball quotes of all time is attributed to famed sportswriter Red Smith. There have been various versions used by Smith, but it essentially goes like this:

Ninety feet between bases is perhaps as close as man has ever come to perfection.

The problem is that the distance between the bases isn't 90 feet at all. What is the 90-foot distance all about? Well, the key is that a 90-foot square (generally called a "diamond") is used to help lay out the bases on the infield. But the bases aren't all the same size (home is a very special shape) and they are not all placed in similar locations relative to the corners of the 90-foot diamond.

In a prior blog entry (which I encourage the reader to review), I discuss the little-known fact that the rules of baseball require that home, first, and third bases each nestle neatly in their respective corners of the 90-foot diamond, but second base is centered on its corner of the diamond.

Here's a diagram (not drawn to scale) from the official rule book that shows the situation:



So, while the infield is laid out on a 90-foot diamond, the shortest distance between consecutive bases is clearly less than 90 feet.

So what are the distances between bases? To answer this question we first need to first define these distances as the shortest length between one base and the next.

It seems that we have four distances to determine: home to first, first to second, second to third, and third to home. However, the layout of the bases is reflectively symmetrical. That is, one can draw a line running through the center of home base and through the center of second base such that the left side and right side of the infield are mirror images of one another. Thus, the distance between home and first is identical to the distance between third and home. And the distance between first and second is the same as the distance between second and third. So, there are actually only two distances to calculate, not four.

Let's calculate two distances that are often covered by base stealers: first to second and third to home. Obviously the dynamics of stealing second base are quite different from those of stealing home, but still it should be interesting to compare the actual distances covered.

The easiest distance to calculate is that between first and second base. The shortest distance between first and second is represented by the double-headed red arrow in the diagram below, while the double-headed blue arrow is identical in length to one side of the 90-foot diamond:



All one needs to know to determine the length of the double-headed red arrow is the size of first and second base (which, of course, is also the size of third base). Each base is a square, 15 inches on a side. So, we need to subtract the full length of first base and half the length of second base (remember it is centered on its corner of the diamond) from the 90 foot double-headed blue arrow to determine the length of the double-headed red arrow:

distance between first and second = 90 feet - 15 inches - 7.5 inches
distance between first and second = 90 feet - 22.5 inches
distance between first and second = 90 feet - 1.875 feet
distance between first and second = 88.125 feet or 88 feet 1.5 inches

Now we are left with the more difficult calculation: the distance between third and home. The problem is the rather strange shape of home base. First, a bit of an aside to explain why it is that we have such an awkward-looking, five-sided home base.

For a number of years prior to the turn of the century, home base was a square, 12 inches to a side. Like first and third, home was nestled snugly in its corner of the diamond. However, in 1900 home plate was changed to its modern shape. Spalding's Official Base Ball Guide of 1900 explained the reasons behind the change:

With the plate placed in accordance with the form of the diamond field, that is, with its corner facing the pitcher instead of one of its sides, a width of 17 inches was presented for the pitcher to throw the ball over instead of 12 inches, the width of each side of the base. But this left the pitcher handicapped by having to "cut the corners" as it is called, besides which the umpire, in judging called balls and strikes, found it difficult to judge the "cut the corner" balls. To obviate this difficulty, the Committee [of Rules], while keeping the square plate in its old place—touching the lines of the diamond on two of its sides—gave it a new form in its fronting the pitcher, by making the front square with its width of 17 inches, the same as from corner to corner, from foul line to foul line. The change made is undoubtedly an advantage alike to the pitcher and umpire, as it enables the pitcher to see the width of base he has to throw the ball over better than before, and the umpire can judge called balls and strikes with less difficulty.

Now back to the calculation. In the diagram below, the shortest distance between third and home is represented by the double-headed red arrow. Note that this double-headed arrow runs from the home-base side of third to the closest corner of home plate. As in the above diagram, the length of the double-headed blue arrow is 90 feet.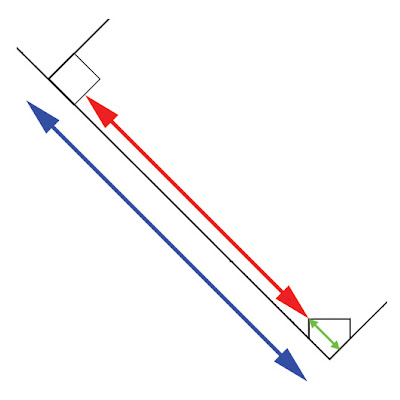



Now for the hard part. What is the distance represented be the double-headed green arrow? The following diagram should help us determine that important information:



Distance C is what we are trying to determine. But C is the sum of distance A and B. To calculate A and B, we simply need to apply the Pythagorean Theorem. Remember that? Here's a refresher: In a right-angled triangle, the square of the hypotenuse (the longest side of the triangle) is equal to the sum of the squares of the other two sides.

By the way, have you ever noticed that near the end of The Wizard of Oz, the Scarecrow, in an effort to show off his new honorary degree of Th.D. (Dr. of Thinkology), incorrectly states the Theorem? His butchered version is "The sum of the square roots of any two sides of an isosceles triangle is equal to the square root of the remaining side." Check it out:



But we've stalled long enough. Back to the math. First let's calculate distance A:

A² + A2 = (17 inches)²
2A² = 289 inches²
A² = 144.5 inches²
A = 12.02 inches

Now distance B:

B² + B² = (8.5 inches)²
2B² = 72.25 inches²
B² = 36.125 inches²
B = 6.01 inches

And so the double-headed green arrow (C) can now be calculated:

C = A + B
C = 12.02 inches + 6.01 inches
C = 18.03 inches

All that is left to do is to subtract the full length of third base (15 inches) and distance C (18.03 inches) from the 90 foot double-headed blue arrow to determine the length of the double-headed red arrow:

distance between third and home = 90 feet - 15 inches - 18.03 inches
distance between third and home = 90 feet - 33.03 inches
distance between third and home = 90 feet - 2.7525 feet
distance between third and home = 87.2475 feet or 87 feet 2.97 inches

Finally, let's compare the two distances between bases:

distance between first and second = 88.125 feet or 88 feet 1.5 inches
distance between third and home = 87.2475 feet or 87 feet 2.97 inches
difference = 0.8775 feet or 10.53 inches

And so we have our answer. Indeed, the distance between bases is very different. In fact, the distance between third and home is over 10½ inches shorter than the distance between first and second.

Sorry, Mr. Smith.

George Bignell

Ever heard of George Bignell?

No? Maybe this will help: Bignell played four games for the old Milwaukee Brewers of the Union Association in 1884.

Still doesn't ring a bell? On October 3, 1884, Bignell set the record for most chances accepted in a game by a catcher with 23. The record has never been equaled.

Now got him?

No? Well, me either. Before I started researching him, I had never heard of the man. Of course, this raises the question: Why research him?

The answer: Because I recently ran across this photo at the New York Public Library's Digital Gallery web site:


New York Public Library Digital Gallery, Image ID: 55702

The photograph is identified as "George Brynan" and is listed under the subject of "baseball." But a quick check of any baseball encyclopedia will reveal that just one person named Brynan ever played in the big leagues: Charles Ruley "Tod" Brynan. Indeed, there doesn't appear to have been a "George Brynan" who played at any level of professional baseball.

Like Bignell, Tod Brynan played just four games in his major league career, pitching in the National League for Chicago (three games in 1888) and Boston (one game in 1891). Coincidentally, an image of Tod Brynan is also available at the New York Public Library's Digital Gallery web site. Alas, the NYPL has misidentified Tod Brynan as George Brynan. Here is that image:


New York Public Library Digital Gallery, Image ID: 55700

Note that at the bottom of the photograph is written "C. BRYNAN. P, CHICAGO." The image is actually of an Old Judge tobacco card of Brynan issued in 1888, part of what is referred to in the card collecting hobby as the N173 set. A similar card was sold at Legendary Auctions in August of 2010 for a whopping $1,777.50.

While there is some similarity between Tod Brynan in the N173 photo and the player in the head-and-shoulders photograph, a more obvious clue caused me to question the identification of the latter image. Take a look at the reverse of that photo portrait:


New York Public Library Digital Gallery, Image ID: 55702

Below and to the right of the New York Public Library stamp is a handwritten name: George Bignall. Below that name is a notation that appears to be an attempt to decipher the handwritten last name ... a rather poor attempt: Brynan. While the latter name was added to the NYPL database, it was the former name that I thought was more likely to be correct. I failed to find a big leaguer named Bignall, but big leaguer George Bignell was pretty close.

The next step was to contact the Pictorial History Committee (PHC) of the Society for American Baseball Research. The Committee's Player Image Index project is an attempt to catalog at least one image of every major league ballplayer. The entry for George Bignell was supplied by ace baseball researcher and New England baseball expert Bob Richardson, who found the following woodcut in the October 10, 1885 issue of the Brockton Gazette of Brockton, Massachusetts:



The image above was clearly based on the photo found at the New York Public Library's Digital Gallery web site. Additionally, the Bignell photo was taken at Bass Photo Studio in Brockton, while the woodcut was published in the Brockton Gazette.

There's really no question: the NYPL photo identified as George Brynan is actually one of former major league catcher George Bignell.

The Wild West at West Side Park

From the mid-1890s to 1915, the Chicago Cubs (known as the White Stockings during much of the 19th century) played their home games at West Side Park, located at the corner of Polk and Lincoln (now Wolcott) Streets. This was the park of the famed Tinker-to-Evers-to-Chance Cubs; the park of the dominant Cubs squad that won an astonishing 116 games in 1906 (they lost just 36); the park that hosted three out of four straight World Series (1906-1908 and 1910), with the Cubbies winning two of those championships.

But beginning in 1916, the Cubs moved to a different home: Weeghman Field (now known as Wrigley Field). With the departure of the Cubs, West Side Park dropped from the major league scene, becoming a venue for semi-pro baseball, amateur games, and (as we shall see) other events.

Thankfully for the baseball researcher, the park is well-documented online, with many hundreds of photos available at the Library of Congress's Photographs from the Chicago Daily News web site. A few of these images follow.

Here's a shot of the park in 1908:


SDN-007231, Chicago Daily News negatives collection, Chicago History Museum

And here is a photograph taken in 1907 at West Side Park showing Giants catcher Roger Bresnahan donning the innovative shinguards he first introduced at the beginning of the season:


SDN-053191, Chicago Daily News negatives collection, Chicago History Museum

And check out this great photo of Pirates legend Honus Wagner at West Side Park. Note that Honus is wearing his cap backwards, a common practice today, but very rarely done a century ago.


SDN-054782, Chicago Daily News negatives collection, Chicago History Museum

As a brief aside, for what it's worth, the earliest example I've found of a baseball player wearing his cap backwards is this 1879 photo of the University of Pennsylvania baseball team:


University of Pennsylvania Photograph Collection Record ID 20050308013

Check out the player standing third from right, one Gustavus Remak:



But let us return to West Side Park in the 20th century.
One of the most intriguing Chicago Daily News photographs taken at the park is this image:


DN-0066934, Chicago Daily News negatives collection, Chicago History Museum

Sure, some great games were played at West Side Park over the years. But none could match the game seen here. Horses, cows and bulls are all over the field and in front of the outfield walls are enormous pieces of scenery, painted to look like mountains. What's the story?

An easy clue is found at the top of the photo: a date stamp seen in reverse. That date is August 23, 1916. What happened that day at West Side Park? Well, take a look at this advertisement from the Chicago Daily Tribune of August 19, 1916:



From August 19 to 27, 1916, West Side Park was the site of a round-up and "Shan-Kive" (purportedly an Indian word for "good time") with "88,000 square feet of scenery": the giant murals in the outfield.

The advertisement noted that there will be "2 complete exhibitions daily at 2:00 and 8:00 p.m." with "15,000,000 candle power illumination." Take another look at the photo above. Could those be small light standards mounted atop the grandstand roof down what once was the left field line?

Additionally, the advertisement states that "all contests [will be] supervised by Col. W.F. Cody (Buffalo Bill)." The 70-year-old Cody was a fixture at the event, one of the last appearances of his illustrious career, as he passed away less than half a year later. Indeed, two other photos from the Chicago Daily News captured the colorful showman seated on the field at West Side Park. Take a look at this wonderful pair of portraits of the famed "Buffalo Bill" taken on August 23, 1916 (and be sure to take note of the stunning mountain scenes in the background):


DN-0066931, Chicago Daily News negatives collection, Chicago History Museum


DN-0066930, Chicago Daily News negatives collection, Chicago History Museum

A promotional description of the event was published in the Chicago Daily Tribune of August 14, 1916. It reads:

OLD CUBS PARK CHANGED INTO "WILD WEST" SCENE.

Arena Being Transformed Into Miniature Wyoming Ranch with Background of Mountains

The cowboys, the cowgirls, and all the other ranch people who are coming to Chicago to contribute to the strenuous incitements of the big Shan-Kive and Roundup, which opens next Saturday afternoon, will find themselves in a familiar scenic atmosphere at the old Cubs' west side ball park.

The work of transforming the arena into a miniature Wyoming ranch, with its panoramic background of hills and mountains, has been rapidly progressing. The scenic effects at the ball park are expected to prove a revelation.

The money prizes, which will be awarded by a board of judges led by Col. William F.Cody, the famous scout and Indian fighter, will range from $1,000 in gold for the "best all round cowboy or cowgirl" to $500 for the winner in a wild horse race. Every day will have its own special contests. Interspersed will be arenic performances by Indians, Siberian Cossacks, Bedouin Arabs, Japanese, and Mexican Vaqueros.

The Cubs may have left months before, but in August of 1916, Buffalo Bill and the Wild West came to West Side Park.